
output) this is where the activation of the current layer is given as input to the derivative of the sigmoid function used as an activation function. However, when it comes to However, when it comes to big data, an individual usually struggles to quickly find the local or global As we go toward this edge the second derivative gets very high and then crosses 0 and goes negative and then goes back to 0, so basically by laplacian on edges we are walking on 2nd derivative of sigmoid, which is shown in Fig.
Derivative of sigmoid function is unimodal with maximum at tinf.

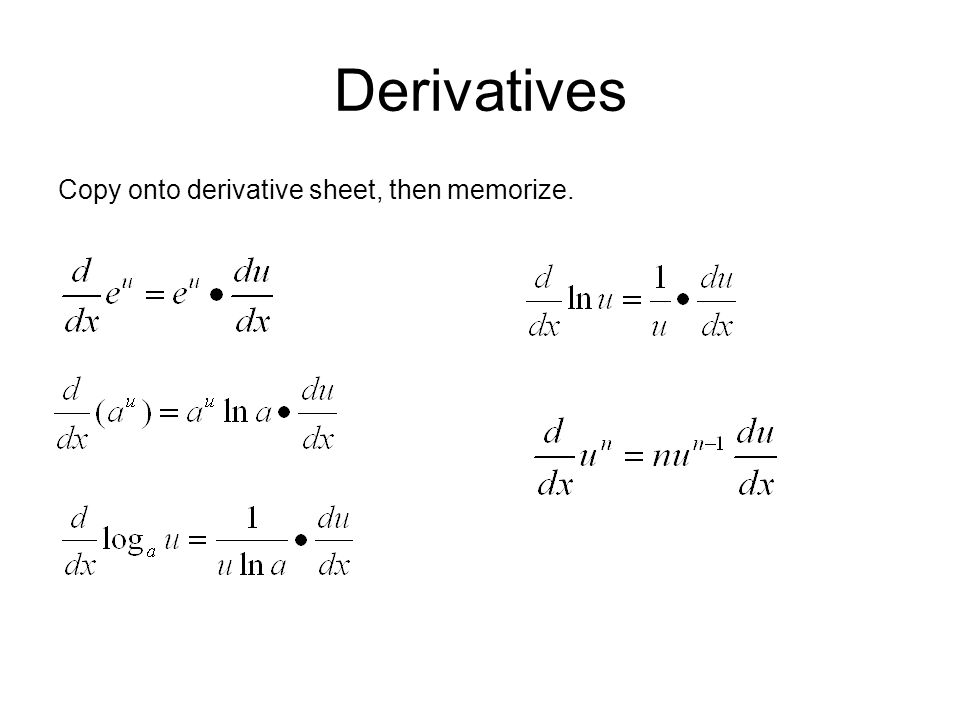
#DERIVATIVE OF LOG U LICENSE#
Textbook content produced by OpenStax is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike License 4.0 license.Second derivative of sigmoid I recall that in the 1990s, it was common advice to use target values in the set of ^N \left ( - y^i \log (\sigma (\theta^T x^i + \theta_0)) - (1-y^i) \log (1-\sigma (\theta^T Derivatives with respect to vectors Let x ∈ Rn (a column vector) and let f : Rn → R.

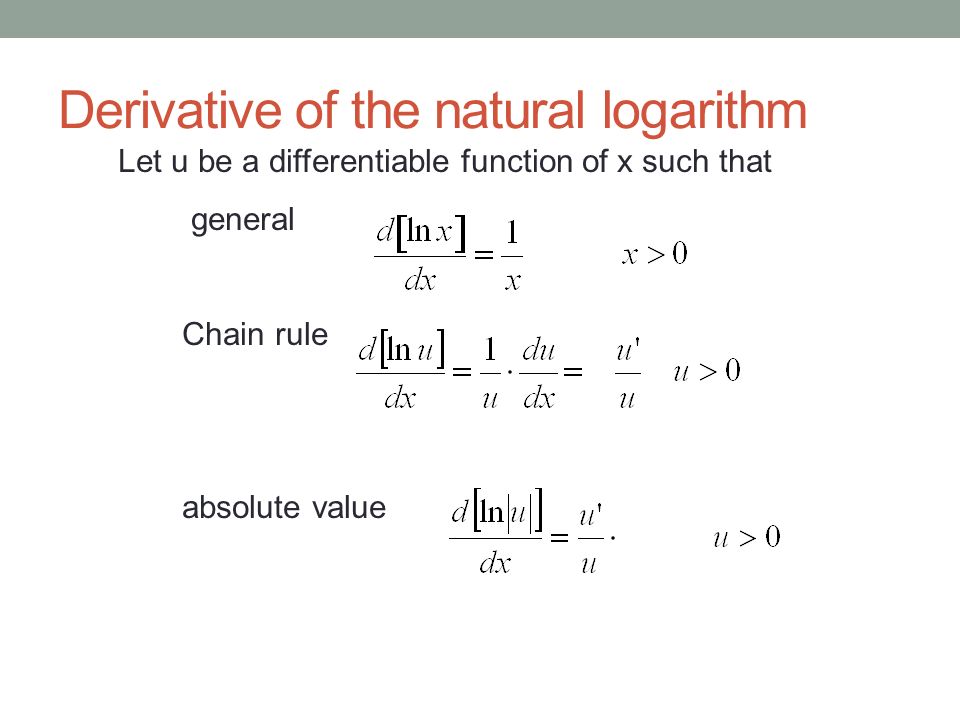
We recommend using aĪuthors: Gilbert Strang, Edwin “Jed” Herman Use the information below to generate a citation. Then you must include on every digital page view the following attribution: If you are redistributing all or part of this book in a digital format, Then you must include on every physical page the following attribution: If you are redistributing all or part of this book in a print format, Want to cite, share, or modify this book? This book isĬreative Commons Attribution-NonCommercial-ShareAlike License 4.0 From this definition, we derive differentiation formulas, define the number e, e, and expand these concepts to logarithms and exponential functions of any base. This definition forms the foundation for the section. We begin the section by defining the natural logarithm in terms of an integral. By the end of the section, we will have studied these concepts in a mathematically rigorous way (and we will see they are consistent with the concepts we learned earlier). We now have the tools to deal with these concepts in a more mathematically rigorous way, and we do so in this section.įor purposes of this section, assume we have not yet defined the natural logarithm, the number e, or any of the integration and differentiation formulas associated with these functions. The definition of the number e is another area where the previous development was somewhat incomplete.
#DERIVATIVE OF LOG U HOW TO#
For example, we did not study how to treat exponential functions with exponents that are irrational. However, we glossed over some key details in the previous discussions. We already examined exponential functions and logarithms in earlier chapters.


 0 kommentar(er)
0 kommentar(er)
